Beta Distribution
The Beta distribution is a very versatile function which can be used to model probability density curves of several different shapes, such as those shown below.
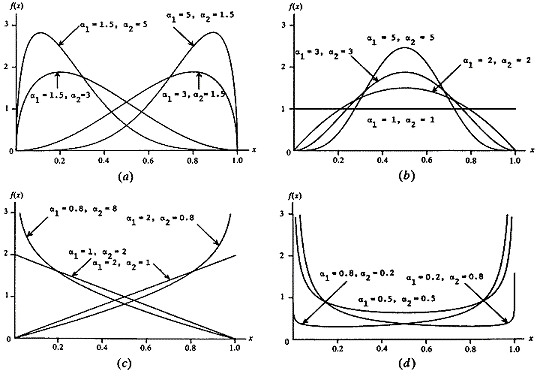
The form of the Beta distribution is determined by the shape parameters a1 and a2. Both a1 and a2 are always positive (greater than 0). The relationship between the shape parameters of the Beta distribution and the RocTunnel3 input data is as follows:
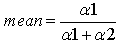
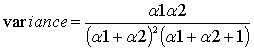
The standard deviation is the positive square root of the variance.
The above equations apply to a beta random variable on [0,1]. To rescale and relocate to obtain a beta random variable on [a, b] of the same shape, use the transformation a + (b–a)X.