‘Open Secret’ Advantages of the Shear Strength Reduction Approach in Slope Stability Analysis
by Reginald Hammah, Ph.D.
Introduction
Seventeen years ago, in 2004, we wrote a RocNews article outlining our perspectives on the Shear Strength Reduction (SSR) technique of slope stability analysis. As you know, this technique allows numerical methods, such as the finite element method (FEM), to determine the factor of safety of a slope.
The article stated that “By examining the merits of finite element analysis and limit-equilibrium methods for slope design and analysis, it is our opinion that, for the foreseeable future, these two approaches will coexist. Together, they supply engineers with a more complete toolkit for tackling slope stability problems. The experience, amassed by the geotechnical engineering profession over the decades, with limit equilibrium methods is invaluable and cannot be readily displaced.
On the other hand, several types of geotechnical problems are not readily analyzed with limit equilibrium methods but can be handled by the SSR FE approach. Today’s technological advances, combined with the reduced costs of computing power, enable the SSR technique to solve these problems easily. In addition, the SSR technique can be used to resolve ambiguities in limit-equilibrium slope stability analysis.”
We were correct that the SSR method would coexist alongside limit equilibrium analysis and that traditional limit equilibrium methods would not be readily displaced. We were hoping, though, that by now, geotechnical engineers would use SSR analysis just as often as they perform limit equilibrium assessments. That has not happened yet. But we believe the time is ripe for this to happen. These are our reasons.
Stress Distribution and Speed (in some cases) – Two Key Challenges of Limit Equilibrium Methods
Determining the critical failure surface or failure mechanism of a slope and the associated safety factor are fundamental tasks of slope stability analysis. In the computer era, limit equilibrium methods have been the most popular for tackling these tasks. And they remain beneficial. However, a lot has also been written about their limitations and the dangers of applying them beyond their limits.
One of the main drawbacks of limit equilibrium analysis is the validity of force distributions. These methods use simplified assumptions regarding the type and magnitude of the internal and external forces acting on slices to make slope stability problems statically determinate. Typically, they limit forces to the weights of slices, arbitrary side forces between adjacent slices, and shear forces along slice bases. Since these assumptions do not consider the relationship between stresses and displacements, they do not always produce realistic stress distributions.
It seems well-accepted that limit-equilibrium methods that produce realistic stress distributions are to be more trusted. (This is particularly true of those that satisfy complete static equilibrium.) Morgenstern and Price, in support of this idea, advocated accepting “physically acceptable” solutions with no tension and containing thrust lines within sliding masses.
Limit equilibrium methods enjoy wide usage partly because of their fast computational speeds. However, in complex geometry problems, it sometimes becomes necessary to increase the number of slices to capture critical failure mechanisms correctly. This can drastically slow down computational speeds and put them even behind the speeds of SSR analysis.
Five ‘Open Secret’ Advantages of the SSR Method
The SSR technique has several open ‘secrets.’ We will outline five key ones.
- It enjoys a substantial advantage over-limit equilibrium methods in the generation of realistic stress distributions. Numerical methods inherently accommodate stress-strain relationships and avoid arbitrary assumptions regarding inter-slice forces.
- SSR analysis does not require a priori assumptions on failure surface types, shapes, and location. Rather, it automatically establishes critical failure mechanisms. The method can automatically monitor the development of failure zones, ranging from localized instabilities to total slope collapse.
- Given realistic deformation properties of materials (Young’s moduli in particular), the SSR method can predict expected deformations at failure. Although deformation properties may not change safety factor values by much, they can alter failure mechanisms. This aspect can significantly improve our ability to support and monitor slopes.
- The SSR technique can accommodate both the peak and residual strengths of materials in the same analysis. This feature much better captures the real-world behaviour of geological materials.
- As alluded to earlier, SSR analysis has a bonus; it can be just as fast or faster than limit equilibrium analysis under some conditions. This typically happens when the number of slices is increased (to capture failure mechanisms accurately).
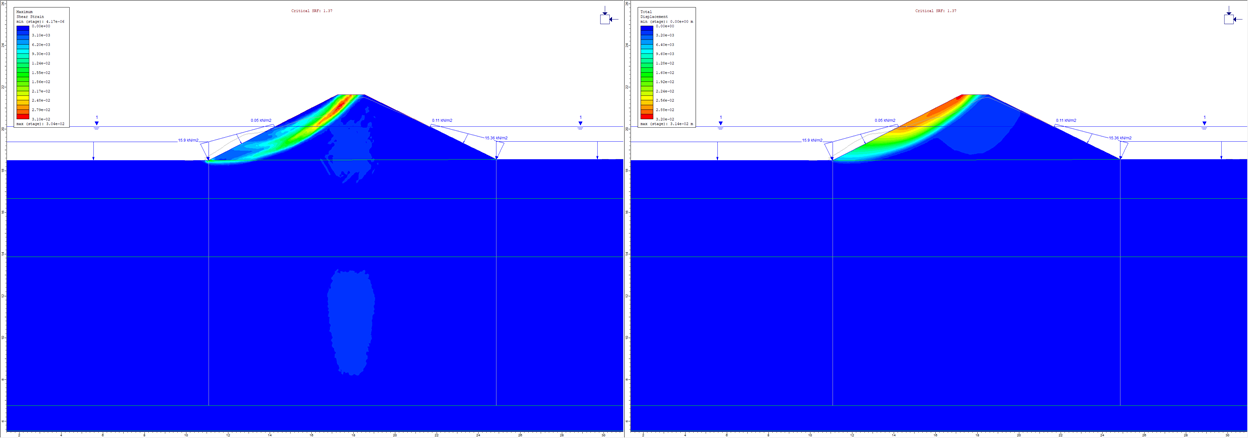
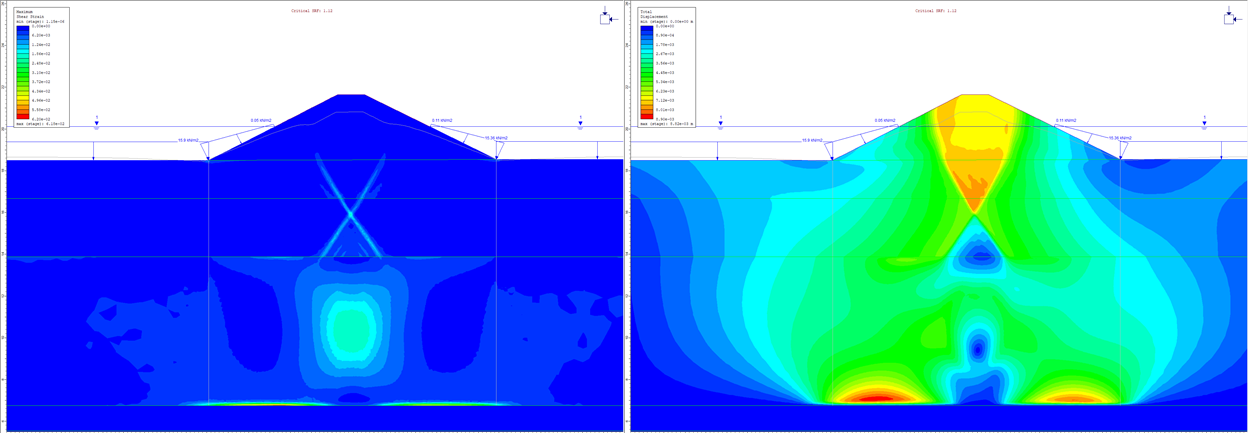
Final Thoughts
Although SSR analysis is not the panacea to every slope stability problem, it offers advantages that must place it, at a minimum, on par with traditional limit equilibrium methods. We hope that the points briefly outlined above convince you to apply the approach much more regularly.
To facilitate the regular application of the method, Rocscience will integrate the SSR method more closely into the limit equilibrium methods. Plans are underway to implement an option in Slide2 for selecting the SSR method in addition to the limit equilibrium methods of slices. When selected, Slide2 will automatically generate an SSR equivalent of a slope for computation in RS2. Slide2 will also be able to display SSR results once the calculations are completed.
We look forward to revisiting this topic in another few years to see how far we have come as an industry with SSR analysis.