Full and Quasi-Stochastic Slope Stability Analysis Using the Random Limit Equilibrium Method (RLEM)
Check out this preview of a paper that will be presented by Dr. Sina Javankhoshdel at GeoCongress 2020 taking place in Minneapolis, Minnesota on February 25-28.
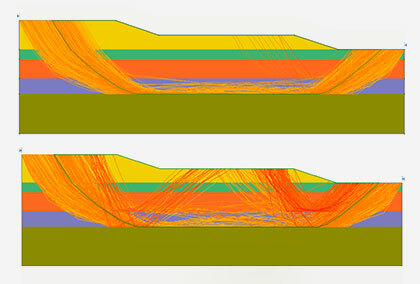
Natural alluvial deposits exhibit variability in strength and stiffness parameters, which results in uncertainty in the response of geotechnical structures such as slopes. The Random Limit Equilibrium Method (RLEM) developed in Rocscience Slide2 offers a conceptually simple, versatile, and efficient approach for the evaluation of the stability conditions of heterogeneous slopes. Limit Equilibrium Methods (LEM) using non-circular slip geometries have proven more accurate than circular slip geometries in profiling the failure status of heterogeneous slopes by passing through the weak points more effectively.
In this study, the influence of considering two-dimensional spatial variability of soil properties on output results is investigated using the non-circular RLEM approach. The results are also compared to 1D spatial variability analysis, which considers spatial variability only along the critical slip surface of the slope. Also, the non-circular RLEM approach results are compared with the circular RLEM approach results for different limit equilibrium methods. Finally, cross-correlation between soil strength parameters is also investigated.
In this study, the deterministic factor of safety calculated using the non-circular LEM method in Slide2 software is 1.3.
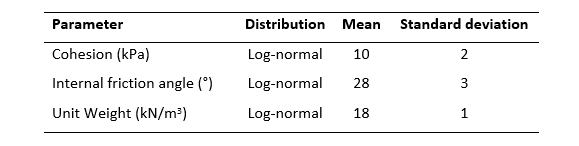
The parameters presented in Table 1 are used in the probabilistic analysis of this study:
Here are some of the concluding remarks presented in this study:
- Consideration of the non-circular slip surface, due to its versatility in capturing arbitrary failure geometries, proved to be more efficient in detecting the most critical stability conditions in comparison to the traditional slice-based circular algorithms. It was shown that the non-circular assumption yields lower factors of safety for the heterogeneous slopes in an average sense. Overall, the reliability index of the nonhomogeneous slopes, considering the non-circular failure geometry, was indicated to decrease the reliability index of the slope by yielding a higher probability of failure.
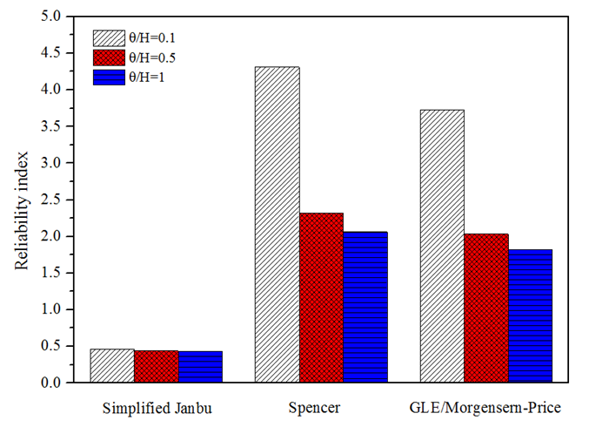
- Neglecting the auto-correlation of the strength parameters in non-circular RLEM would render unconservative results as supported by the reliability charts for different scales of fluctuation (Figure 1).
- The strength parameters’ auto-correlation structure was shown to have a serious reliability impact on slope stability analyses through adoption of the RLEM approaches. Disregarding the correlation structure of the strength parameters, namely the cohesion and the internal friction angle, leads to increased reliability indices and therefore an unconservative stability evaluation to be given out (Figure 2).

For a full discussion of the study, join Dr. Javankhoshdel at a special GeoCongress 2020 session on Recent Advances in Risk-Informed Geotechnical Design and Management on Wednesday, February 26, 2020.